The low-risk anomaly in large emerging markets

The Capital Asset Pricing Model (CAPM) assumes a positive risk-return relationship. This positive risk- return relationship has been questioned and rejected in literature due to the existence of other persistent factor premia. One such factor premium is the premium that low-volatility stocks earn in the market. In this empirical study, we will apply factor models in order to prove that the low-volatility anomaly persists in emerging stock markets.
Introduction
The low-risk anomaly states that on average low-volatility stocks tend to outperform high-volatility stocks. Behavioral finance attributes the anomaly to irrationality of investors and the benchmarking of institutional investors. This anomaly persists across time, across different risk measures, across industries and markets and after controlling for size, value and momentum. This study examines the presence of the low-volatility anomaly in large emerging markets. The added value of this study is the following. First, we add to the evidence on the anomaly in emerging markets. Secondly, our sample allows for an in-depth country-specific analysis. Finally, our results advocate for a factor-based investing approach and give a possible way to construct low-volatility portfolios to profit from the low-volatility anomaly.
More precisely, we examine the anomaly using total return index information from South Korea, Taiwan and Brazil for a 21-year period (31/12/1998-31/12/2019). These three countries are part of the investable MSCI EM index. Total return indices and market capitalization information are extracted from the Thomson Reuters Datastream database and from the Kenneth R. French data library and afterwards used in factor models.
Methodology
We sort the stocks into five quintiles (Q) based on trailing total volatility of daily returns. The stocks with the lowest trailing volatility go into Q1, stocks with the highest trailing volatility go into Q5. Following our hypothesis, the low-volatility stocks should outperform high-volatility stocks. This implies that we hypothesize that the Q1 alphas will be positive and statistically significant, whereas the Q5 alphas will be negative and statistically significant.
We formally test our hypothesis by regressing monthly excess portfolio returns in three different factor models, namely the CAPM, the Fama French Three-Factor model and the Carhart Four-Factor model. Throughout the regression analysis we will work with simple returns. The excess portfolio returns are calculated in excess of the monthly T-bill rate. As our market portfolio, we use an equally weighted portfolio of all stocks in our stock universe.
Results
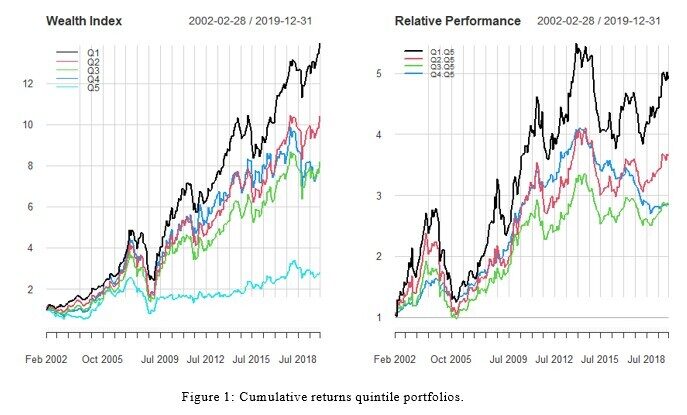
After constructing the quintile portfolios, we also conduct a descriptive analysis on their return distributions. Summary statistics on the quintiles all point to the presence of the anomaly as seen in Figure 1, in which the kurtosis depicted is the excess kurtosis. The relative performance of quintile 5 is inferior to all other quintiles while the standard deviation and variation in returns is larger. Quintile 5 has lower average returns, lower cumulative returns, lower risk-adjusted returns and larger downside. Moreover, quintile 5 has the highest positive skewness. This supports the lottery characteristics rationale. The left panel of Figure 1 shows the wealth indices of the quintiles. It depicts what 1 USD invested at 𝑡0 in each of the five portfolios would appreciate to, assuming all proceeds are reinvested. The right panel shows the relative performance in comparison with the most volatile quintile Q5. Both panels show that higher volatility leads to a decrease in performance.
The regression results in Table 2 (see appendix below) are in favour of our hypothesis and in line with existing finance literature on factor premia. They provide additional empirical evidence on the existence of the anomaly and thus the failure of CAPM. The alphas show a negative relationship between risk and return that in some instances becomes flatter but never positive. Newey-West heteroskedasticity and autocorrelation consistent (HAC) standard errors are given between brackets.
Appendix

Auteurs

